This purpose of this page is to carefully describe the difference between a pure tone--a single frequency sine wave--and a complex tone--a musical note that consists of a number of harmonically related frequencies. Why the big deal about pure and complex tones? Well, as we shall see, musical tones from plucked strings or hollow tubes are complex tones as a natural consequence of how the notes are produced. Complex tones are vital to understanding why we humans find certain pitch intervals especially pleasing (consonant). We'll delve into that topic in more detail when we tackle the subject of the Scientific Theory of Consonance in class and in a later web page. Right now I jaut want you to be able to clearly distinguish the concepts of pure and complex tones.

Lets start with a pure tone. A pure tone is a sound that consists of one single frequency. The sound wave, if we recorded it, would be a perfect sine pattern that repeated over and over with a given repetition rate. A pure tone would be created if a simple harmonic oscillator emitted sound waves. Remember we said that a simple harmonic oscillator had only one resonant frequency. Let's let our senses do the walking--here is a sound file of a 200 Hz pure tone and a plot of the 200 Hz pure tone as a function of time.

Note that you cannot read the frequency directly from the figure; but you can read the PERIOD and hence get the FREQUENCY. Try it to make sure you get the answer of 200 Hz.

Now how about a complex tone. A complex tone is a sound wave that repeats with a given pattern BUT the pattern is not a sine wave. As we will see the complex tone consists not only of one frequency sine wave but rather a number of different frequency sine waves superimposed on top of one another. In the time domain the repeat time of the pattern gives the period of the lowest frequency sine wave that is contained within the complex tone. All of the other sine wave frequencies in the complex tone are integer multiples of this lowest frequency. A complex tone is created by a plucked string or a exciting the modes of a hollow tube. These two systems (string or tube) are the basis of almost melodic instruments. Note that neither system is a simple harmonic oscillator: rather each possesses a sequences of resonances whose frequencies are harmonically related hence they emit complex tones. Some of these ideas will become clearer as we look at the cocept of spectrum. Again lets look at an example, here is a 200 Hz complex tone for your listening and visual enjoyment.

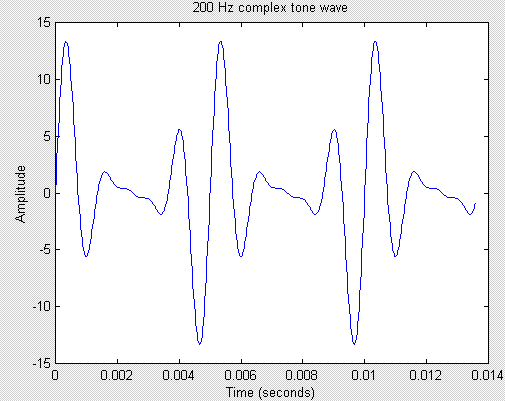
Ok a few questions to finish the lesson:

1. What is the period of the complex tone in the figure above? What is the lowest frequency that makes up this tone? This lowest frequency is called the fundamental of the tone.

2. What other frequencies are possibly in this complex tone? List 4 other frequencies that might be in the tone. [Make sure you understand what integer multiples of the lowest frequency means].

3. For those musically-minded folks: is the pitch of the pure and the complex tones the same? If you say no then which note has the higher pitch? You should know that there is almost no 200 Hz component in the complex tone--the tone is dominated by higher harmonics.

Closing comment (a la Jerry Springer?). Looking at the plot of the complex tone in time gives you some information about what the tone is made up of but it really leaves a lot of open questions. Although we know the fundamental frequency we don't know how much of that frequency is there in the tone and how much is due to the other harmonics. As we will see, the technique of spectral analysis comes riding in to answer all these questions.