Pythagoras, a Greek philosopher from Samos who lived about 569 BC - about 475 BC, made important developments in mathematics, astronomy, and the theory of music. What is called the Pythagorean theorem was known to the Babylonians 1000 years earlier, but Pythagoras may have been the first to prove it.
see http://www-groups.dcs.st-and.ac.uk/~history/Indexes/HistoryTopics.html and go to the Bibliographies Index.

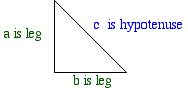
The sum of the squares of the lengths of the legs is equal to the square of the length of the hypotenuse in a right triangle, or, more familiarly,

a² + b² = c²,

where a and b are the lengths of the legs and c is the length of the hypotenuse.

The Pythagorean theorem can be used to find the length of the hypotenuse of a right triangle when one knows the lengths of the two legs and to find the length of one leg when one knows the length of the other leg and the hypotenuse.

When we know the length of the two legs, we can easily find the length of the hypotenuse in this manner:

One leg is 7 feet long and the other is 24 feet long, how long is the hypotenuse?
We set up the item like this: a = 7 and b = 24, find c.

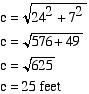
As you see from the example, these problems are best solved using a calculator. Remember to use the radical, ALWAYS put the large number first, and close your parentheses.

Let’s review the key strokes on the calculator to use it for the above example.

[2nd][x²] gives you √( 

then type in the larger of the two numbers and [x²]

[ + ] the smaller number [x²] [ ) ] [Enter]

For this example:

[2nd][x²] [ 2 ] [ 4 ] [x²] [ + ] [ 7 ] [x²] [ ) ] [Enter]

You’ll see on the screen:

√( 24² + 7²)
                                  25

To find the hypotenuse add the squares of the legs.

To find a leg when you know the hypotenuse and the other leg, SUBTRACT the leg squared from the hypotenuse squared, like this:
If the diagonal brace of a tower is 25 feet long and the horizontal span is 24 feet, how high is the vertical leg of the tower?

The diagonal is the hypotenuse and the span is one leg. The vertical is the other leg.
So: c = 25 and b = 24, find a

a = √( 25² - 24²)
a = √( 2625 - 576)
a = √49
a = 7 feet

As you see from the example, these problems are best solved using a calculator. Remember to use the radical, ALWAYS put the large number first, and close your parentheses.

Let’s review the key strokes on the calculator to use it for the above example.

[2nd][x²] gives you √(

then type in the larger of the two numbers and [x²]

[ - ] the smaller number [x²] [ ) ] [Enter]

For this example:

[2nd][x²] [ 2 ] [ 5 ] [x²] [ - ] [ 2 ] [ 4 ] [x²] [ ) ] [Enter]

You’ll see on the screen:

√(25² - 24²)

                                  7